Experiencing Bluetooth® issues with Go Direct® sensors on Chromebooks? Get help here.
Menu

Experiments​

Newton’s Law of Cooling

Experiment #30 from Physics with Vernier

Education Level
High School
College
Subject
Physics

Video Overview

Newton’s Law of Cooling

Introduction

A container of hot water at temperature, T, placed in a room of lower temperature Troom, will result in an exchange of heat from the hot water to the room. The water will eventually cool to the same temperature as the room. You observe this cooling process every time you wait for a hot drink to cool. In this experiment, you will examine the cooling of hot water, with the goal of creating a model that describes the process. You can also predict the time it takes for the hot water to cool to room temperature.

Isaac Newton modeled the cooling process by assuming that the rate at which thermal energy moved from one body to another is proportional (by a constant, k) to the difference in temperature between the two bodies, Tdiff. In the case of a sample of water cooling in room temperature air

{\text{cooling rate}} = -k{T_{diff}}

From this simple assumption, he showed that the temperature change is exponential in time and can be predicted by

T_{diff} = T_{0}e^{-kt}

where T0 is the initial temperature difference. Exponential changes are common in science. Systems in which a rate of change is proportional to the changing quantity show exponential behavior.

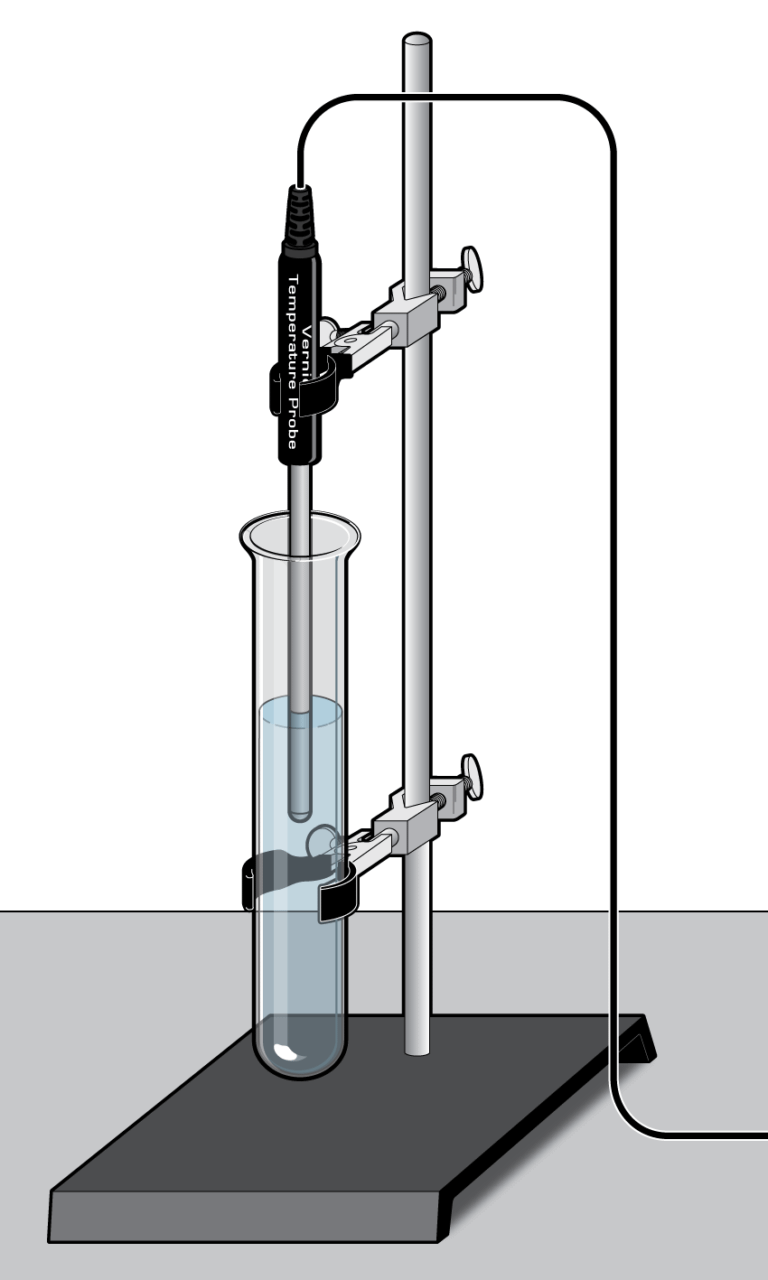
To complete this experiment in a short time, you will use a small quantity of hot water, at a temperature about 30°C above room temperature. A Temperature Probe will record the water’s temperature as it cools.

Objectives

  • Use a Temperature Probe to record the cooling process of hot water.
  • Test Newton’s law of cooling using your collected water temperature data.
  • Use Newton’s law of cooling to predict the temperature of cooling water at any time.

Sensors and Equipment

This experiment features the following sensors and equipment. Additional equipment may be required.

Option 1

Go Direct® Temperature Probe

Option 2

Stainless Steel Temperature Probe

Ready to Experiment?

Ask an Expert

Get answers to your questions about how to teach this experiment with our support team.

Purchase the Lab Book

This experiment is #30 of Physics with Vernier. The experiment in the book includes student instructions as well as instructor information for set up, helpful hints, and sample graphs and data.

Learn More

SAVE/SHARE YOUR CART