Up And Down: Damped Harmonic Motion
Experiment #24 from Real-World Math with Vernier
- Education Level
- High School
Introduction
An object hanging from a spring can bounce up and down in a simple way. The vertical position of the object can be described mathematically in terms of a simple sinusoidal equation. In the real world, however, resistive forces such as friction are always present and cause the object to slow down. This effect is called damping.
Most oscillating objects experience damping and move in a modified periodic manner so that the amplitude gets smaller and smaller with each cycle. Common examples of damped oscillators include an empty rocking chair as it comes to rest after being pushed and a vibrating diving board after a swimmer leaves it. At first, the problem of modeling this type of motion with a mathematical equation may seem extraordinarily complex. Surprisingly, it can be analyzed rather thoroughly using basic math concepts with which you are already familiar.
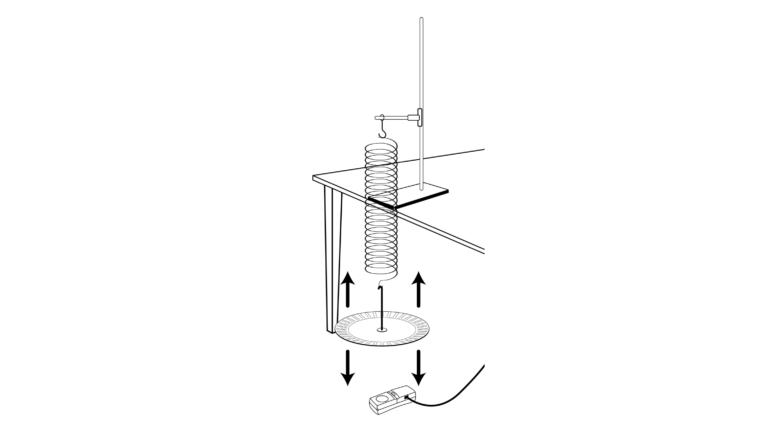
In this activity, you will collect motion data as a paper plate attached to a light spring oscillates up and down above a Motion Detector. Then, you will find an appropriate mathematical model for the resulting data set.
Objectives
- Record the motion data for a plate bouncing at the end of a light spring.
- Analyze the motion data to determine frequency, period and amplitude information.
- Model the oscillatory part of the data using trigonometric functions.
- Model the damping using an exponential function.
- Create a composite model of damping and oscillation.
- Compare the composite model to experimental data.
Sensors and Equipment
This experiment features the following sensors and equipment. Additional equipment may be required.
Option 1

Option 2

Ready to Experiment?
Ask an Expert
Get answers to your questions about how to teach this experiment with our support team.
- Call toll-free: 888-837-6437
- Chat with Us
- Email support@vernier.com
Purchase the Lab Book
This experiment is #24 of Real-World Math with Vernier. The experiment in the book includes student instructions as well as instructor information for set up, helpful hints, and sample graphs and data.

